Apr 18

Apr 18 Fermi Paradox through the Lens of Relativity

Explosion in the Supermassive Black Hole, Sagittarius A*, at Milky Way’s Center (Courtesy of NASA)

“Time is an Illusion”

— Albert Einstein

In my spare time over the past few months, I have been focusing on writing and revising two Outer Space-related legal articles—one of which should be coming out in the next couple of months—yay! But I also want to ensure that I am not neglecting my #TheSpaceBar blog. However, with all that concentration spent on more “formal” legal analysis recently, I thought about turning to something fun—read: not legal-related—about Outer Space. With me always wishing I had more time these past few weeks, the first topic that came to my mind is the spacetime continuum!

As I learned about the space-time continuum, Einstein’s theories of relativity came up in many places. But, these topics are pretty expansive—one blog post can, maybe, barely scratch an atomic part of that surface. So for this post, I will drill into a specific aspect of the spacetime continuum and Einstein’s theories of relativity: gravitational time dilation. After providing a brief background, I will use the lens of relativity to refocus our prior discussion of the Fermi Paradox. Specifically, I will propose how gravitational time dilation can be a fanciful yet fun solution to the Fermi Paradox.

****Quick Note: if I got any of the science below wrong, please do let me know! I self-learned the topic online, so always appreciate a mentor to guide me toward the right cosmic arc 😊****

Einstein’s Theories of Relativity: “The Times They Are A-Changin’”

Einstein’s theories of relativity are made up of two distinct but interconnected theories: special relativity (published in 1905) and general relativity (published 10 years later in 1915). The main difference between the two theories is that special relativity focuses on constant motion (movements that do not change in speed or direction) whereas general relativity focuses on accelerated motion (movements that can change speed or direction). The mathematical proofs for these theories are extremely complicated—at least to me—and entire semesters’ worth of classes can be devoted to every nuance of relativity. So, for the purposes of this post, I am going to focus on explaining a specific aspect of these theories: time.

Special Relativity: The Spacetime Continuum

One of the most significant accomplishments of special relativity is its ability to solve a glaring inconsistency between Newton’s Laws of Motions and the Maxwell’s Equations.

Under Newton’s Laws of Motions, an object’s speed is not absolute and is calculated relative to a specific frame of reference. For instance, while an outside observer at rest might see a blue car traveling at 100 km per hour, the driver of such blue car will not arrive at the same conclusion. To its driver, the blue car does not appear to move at all because the driver is also traveling at 100 km per hour. Furthermore, if there is a red car traveling in the same direction as the blue car but is seen going at 60 km per hour to the outside observer at rest, the driver of the red car would see the blue car traveling away from her at 40 km per hour (the difference in speed between the two cars). So, depending on the frame of reference, the blue car could be (i) moving at 100 km per hour—relative to the outside observer at rest, (ii) traveling at 40 km per hour—relative to the driver of the red car, or (iii) not moving at all—relative to the driver of the blue car. Hence, an object’s speed depends on the observer’s own state of motion.

But under the Maxwell’s Equations, electromagnetic waves (such as light) are determined to have a fixed speed of approximately 300,000 kilometers per second. This speed does not vary according to the observer’s own state of motion. In other words, it doesn’t matter whether the observer of this electromagnetic wave is in motion or at rest, the electromagnetic wave will always be moving at 300,000 kilometers per second.

Thus, an obvious issue arises. Electromagnetic waves would violate Newton’s Laws of Motion because their speed is not relative to the observer’s own state of motion. Therefore, Newton’s Laws of Motion could not always coexist with the Maxwell’s Equations. This would appear to indicate that one of these theories is wrong.

But Einstein was able to fix this inconsistency and unify these two ideas through his Theory of Special Relativity. Under special relativity, Einstein proposed that time itself is not a constant and can move in a relativistic fashion as well. So different observers with different states of motion could experience passage of time that is relatively different between the two. As an observer travels faster and faster through the physical three dimensional space, time would slow down for that individual relative to the passage of time for an individual at rest. By suggesting that time is a variable, electromagnetic waves’ constant speed under the Maxwell’s Equations would no longer violate Newton’s Laws of Motion. This is why light (as a form of electromagnetic wave) would appear to travel at the same speed for both an observer at rest and one in motion. Here, speed is measured in “distance per unit of time” and so a change in “unit of time” can negate a change in “distance.”

As a corollary to this conclusion about time, Einstein essentially demonstrated that the passage of time for a group of individuals can be manipulated—by zooming through the universe. Thus, if we achieve the ability to travel at lightspeed, then we could “slowdown” how fast time moves for those individuals traveling at lightspeed relative to others who are, essentially, staying still on Earth. Therefore, time has essentially become another variable that can be changed alongside the positional variables for the three dimensional space we live in. And henceforth, the concept of “spacetime continuum” was born.

But under special relativity, Einstein did not factor in the effect that gravity might have on the spacetime continuum. This is also why this theory is called special relativity because it would only work in “special” conditions where the effects of gravity can be negated—and, thus, motion would remain constant. However, after 10 more years of thoughts, discussions, and calculations, Einstein was able to build in the effects of gravity in his Theory of General Relativity.

General Relativity: Gravitational Time Dilation

Once Einstein had established the concept of spacetime continuum through special relativity, he then went on to consider the effects that gravitational forces could have on this fabric. In 1915, he published his conclusions on this topic in a series of papers that formed the foundation for his Theory of General Relativity. Under general relativity, Einstein found that an object—via its gravitational force—can warp the spacetime continuum around it. Thus, every single object (stars, planets, pulsars, blackholes, and etc.) in the universe is continuously curving the fabric of spacetime around it. The amount of influence an object can have on its surrounding spacetime environment is dependent on its mass. The more massive an object is, the stronger its gravitational force will be. The higher its gravitational force, the more the object can warp the spacetime continuum around it.

As the curvature in spacetime increases, the passage of time will become slower in those regions relative to farther away regions that are less affected or not affected by the object’s gravitational force. This is because time, as another variable in the four dimensional spacetime continuum, is now “traveling” along the curvature length which is longer than the original linear length. Hence, this will cause a differential in the passage of time between the two positions. The person who is experiencing a higher “degree” of gravity will appear to move through time slower—in relative terms—than the person who is experiencing a lower “degree” of gravity.

Additionally, because the magnitude of an object’s gravitational force increases as you move closer to the object’s center, that means the effect on the spacetime continuum becomes more pronounced as you get closer to the center. So while a person, Alice, residing on the surface of a planet would experience the passage of time the same way another person, Bob, would living on the planet’s tallest mountaintop, in relative terms, Bob would be aging and moving through time faster than Alice.

If there is a huge disparity between the gravitational forces of two positions, then the difference in the passage of time between these two points could become consequential. This could be as significant as how the passage of time can differ under special relativity for a person traveling at lightspeed relative to a person essentially standing still on Earth. This “manipulation” of time via the warping of the spacetime continuum by an object’s gravitational force became known as the concept of “gravitational time dilation”—most famously represented in the movie Interstellar.

Resolving Fermi Paradox through Gravitational Time Dilation

Now that you are armed with this basic understanding of gravitational time dilation, I will now discuss why this concept could be an explanation—albeit farfetched one—to the Fermi Paradox.

As you will recall from one of my recent posts, the Fermi Paradox is a question that has puzzled generations of scientists. Proposed by Italian scientist Enrico Fermi, the issue centers around the fact that with so much evidence pointing to the existence of extraterrestrial intelligent life, why have we not encountered any signs of another intelligent civilization? Well, the theory of general relativity could provide another solution to the Fermi problem: it is because these alien civilizations are moving through the spacetime continuum on a much slower time scale than humanity is.

So while humanity and this other intelligent alien civilization might exist in the same three dimensional space, there could be a huge disparity in the last dimension of the continuum: time. This difference would be caused by the alien civilization experiencing a much greater gravitational pull than that experienced by humanity, causing a significant gravitational time dilation. This difference in timescale could be so significant such that when humanity has already experienced the passage of a few years, these aliens might have only experienced the passage of a few days or hours. So even if the extraterrestrial civilization is developing at the same rate that humanity is developing, the two civilizations would be blissfully ignorant of each other because the relative timescale between the two are completely off. With this intelligent civilization moving at what seems like a “snail’s pace” to us, it would take many more turns of the hourglass before humanity would be able to pick up remote traces of this extraterrestrial life.

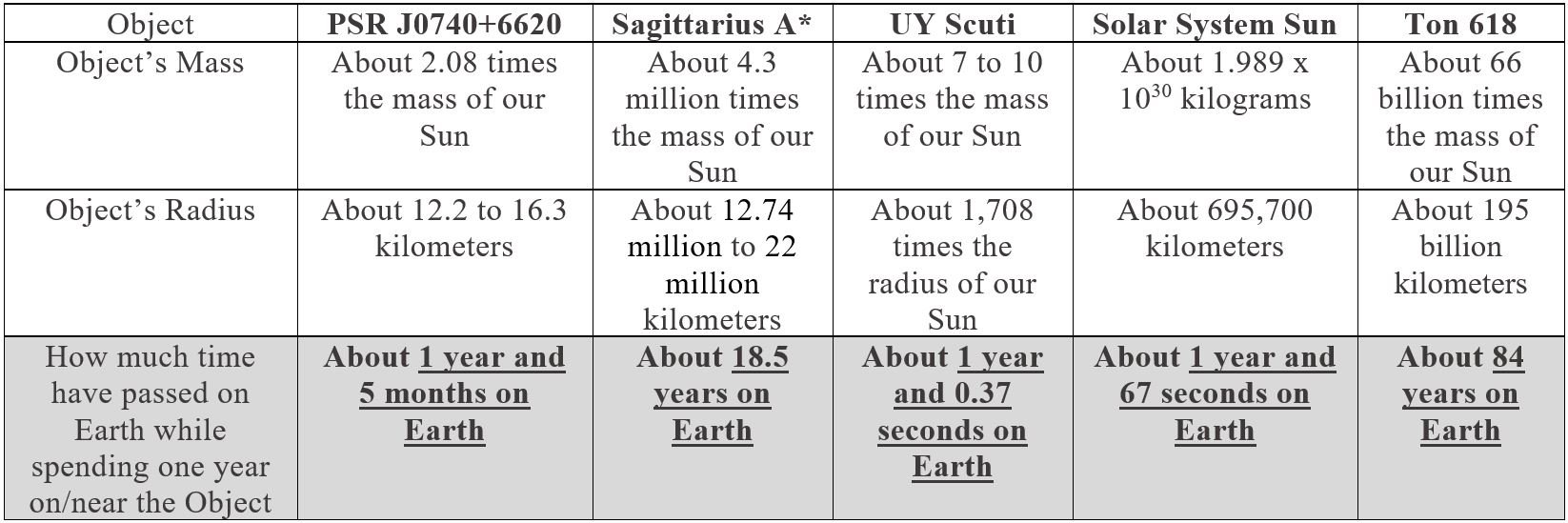
But under our current physical understanding of the universe, this is likely a fanciful solution. The main issue is that such a huge difference in time scale can only be possible if this alien civilization is in a region that is experiencing significantly more gravitational forces than what we are experiencing. The only feasible solution would be for the alien civilization to be close to an enormously massive object, a black hole or a super dense object, a neutron star. Then taking this case to our Milky Way Galaxy, the most likely candidates are the supermassive black hole at our galaxy’s center: Sagittarius A* and the neutron star: PSR J0740+6620. With these objects and their properties identified, we can use the gravitational time dilation calculator to figure out the possible differences in timescale between the two civilizations. Table One below lists these results. For good measure, I also included the differential to Earth’s timescale from (1) our Sun, (2) the largest known blackhole in the universe—Ton 618, and (3) the largest known star—UY Scuti.

Table One: Timescale Differentials* to that of Earth for Selected Objects in the Universe

*Calculations for each are available here: PSR J0740+6620 Calculations (using 12.2 kilometers for radius), Sagittarius A* Calculations (using 12.74 million kilometers for radius), UY Scuti Calculations (using 10 solar masses), Sun Calculations, and Ton 618 Calculations.

Looking at Table One’s results, let’s focus on locations in the Milky Way: the sun, the neutron star PSR J0740+6620, and the supermassive black hole Sagittarius A*. Relative to Earth, the difference in passage of time near the Sun is not that significant; you are just going to age about 67 Earth seconds slower per year on the Sun. However, this is still a higher differential in timescale when compared to that near UY Scuti, where you would only age less than an Earth second slower per year. Next, if you are exploring the neutron star—PSR J0740+6620—for a year, it would be the equivalent of someone else spending one year and five months on Earth. While it is nice to age about five months relatively slower than everyone else, in a decade, you would only be younger by a little bit more than four Earth years; under the cosmic timeline, this is also pretty insignificant.

But, when you look at the timescale difference between Sagittarius A* and Earth, things do get a bit interesting. If an alien civilization is developing near Sagittarius A*, then one year of development there would be equivalent to humanity experiencing 18.5 years of development on Earth. While this is not as significant as the timescale difference between Earth and the region around the supermassive blackhole Ton 618, it is still one order of magnitude different. So now this begs the question: would this be enough evidence to solve the Fermi Paradox?

Well, if we take the origins of humanity as when homo sapiens first came into existence, then human species has been around for about 200,000 years. But, it was only within the last 50 years that we finally broadcast a signal into the Milky Way indicating that there is an intelligent species on Earth. If we were to assume that such an intelligent alien civilization would develop along the same trajectory that humanity has, then it would take the alien race about 3.7 million Earth years (200,000 years x 18.5 timescale) to send a signal out, and about 25,640 more years—the lightyear distance between Sagittarius A* and Earth—for us to receive it. Under these assumptions, given that this alien civilization still has a couple of millions of Earth years to go before they can send out a signal, I guess there is a chance that gravitational time dilation could explain the Fermi Paradox. The rationale would simply be that not enough time has passed for us to become aware of this alien civilization near Sagittarius A*.

But under our current understanding of the universe, this would be a farfetched solution. As humans, we would never be able to survive that environment’s gravitational conditions. Studies have shown that the human body would not be able to sustain anything more than 3 or 4 times Earth’s gravity long-term. Three or four times Earth’s gravity is “just” a few magnitudes less than that of the gravitational forces needed at Sagittarius A* to produce this kind of time dilation to Earth. Also keep in mind, you generally do not want to go anywhere near a blackhole, much less a supermassive one. So it is highly likely that no civilization could develop or settle in that environment, making this a whimsical, albeit fun, solution to think about.

However, our understanding of the universe is constantly changing. Maybe this alien civilization has the type of physiological characteristics needed to survive that kind of environment. After all, “life, uh, finds a way.” With scientists still trying to figure out how supermassive blackholes came about, maybe this alien civilization is the one who created the Sagittarius A* blackhole in the first place. But in that case, I will say that if this is the solution to the Fermi Paradox, then we really need to hope that this is a benevolent alien civilization. Because they would be way more capable of surviving the natural conditions of the galaxy than humanity currently is, and I am not sure if we want to become their enemies.